Should hero bet this flush-completing turn given the high hand bonus implications?

This is an interesting adjustment. I rarely ever adjust for promos other than ones that change the actual money in the pot like a splash the pot or mystery cash envelope, or if I’m at a casino where they do an increase to the bad beat jackpot during certain hours and it starts at 4, I’ll tank two minutes at 3:58 before raising with the second nuts bad beat qualifier.

Ugh, I made a long post here but it disappeared.

AJs and AQs can make more one card effective nut straight flushes, while AKs can only make the royal

AQs makes effective nuts on a board with JT98.

AJs makes effective nuts on boards of QT98 and T987.

In what universe do you semibluff and then all of a sudden wake up once youve hit it on the tuen and think “now i should check”?

Like, the point of a semibluff is to get folds, not to shovel money in when youre behind, so if you dont want folds with a 1 outer to $1k, whats the point of the flop bet other than to value own yourself?

Betting the turn accomplishes something. You gotta decide how often he calls. betting the flop accomplishes nothing. So i guess to me the flop is the big error.

Correct, if hero is aware of the EV implication of the high hand bonus, then they should check the flop as it's clearly the highest EV option.

Checking is also the highest EV option on the turn, for the same reason.

Im sorry but if you lump the turn in with the flop then you dont understand what im saying. You are viewing this as raising the EV of our check. A better way to view this is that it is lowering the value of our fold equity.

On the flop, the ONLY incentive to bet is FE, and without FE, V’s tendencies literally dont matter. You should check the flop whether V is calling with near 100% of their range (because youd be value owning yourself unless they are calling with a range weaker than K high with FD) or 0% of their range (because the equity we gain from folds is negative, since BBJ is worth more than their share of the pot)

On the turn, if V is calling at 100% frequency, obviously we should bet. So you can run your little sims and have a whole discussion about it if you want, but running a sim on this specific turn spot is asinine because the flop bet is a completely objective punt, so we shouldnt have ever been in this situation, and we dont need a supercomputer to figure that out.

Hero's strategical decisions on the flop/turn are measured in EV (not in fold equity). The high hand bonus adds EV to all decisions (betting and checking) but it adds the most EV to checking and as a result makes checking the highest EV option and the clearly dominant strategy. Electing to bet instead is losing EV.

There are many incentives to bet the polarized sections of hero's range on the flop, and of course K♦T♦ is a high-equity semi-bluff that should be included, all else being equal. There's not only fold equity to consider, but also betting balanced ranges, etc. However, the difference in this hand is of course the high hand bonus and it means that this specific combo (and any other straight flush draw) should be checking instead of betting.

It gets kind of murky if you start imagining hypothetical situations where villain is calling/folding 100% of the time, but there's a good argument for betting K♦T♦ on the flop if villain is going to call with 100% of their limp-calling range, as K♦T♦ is a long way ahead of that range. If villain was going to fold 100% of their range to a bet then then EV of betting with any betsize would be the size of the pot on the flop (105) and, as you indicated, checking is a much high EV option as a result (EV around 151.47, thanks in no small part to the high hand bonus) but obviously this is only because hero has a huge draw.

The high hand bonus EV is worth nowhere near as much as hero or villain's equity share of the pot (even on the flop) so the equity hero gains from a fold on the flop/turn would be positive. But that's irrelevant to the fact that checking is still the highest EV option in both cases.

If villain is calling 100% of the time, of course we should bet the turn as the EV would become higher than checking. But villain obviously isn't calling 100% of the time, so it's unclear what point you are trying to make. The EV of hero's choices on the turn are clear from the solve, and checking is clearly the highest EV option.

Why do you claim that the flop bet is a "completely objective punt"?

i mean flop becomes a check for the same reason as the turn no?

idk exactly what hes saying otherwise

think its interesting. would guess at some threshold of high hand equity / bonus it just completely warps the game - similar to how people yell at people that bet instead of checking down bbj hands

if anything flop prob more of whatever the passive option is bc additional ev is worth more of the pot. overall strategy shouldnt change much as we just go the passive route with this type of draw and less often w other ones

sadly u r going to realize people are not here (at this forum) to do the math

Sure, but we had already established that the flop should be a check several posts earlier, so it's unclear why he felt the need to reiterate it. He seems to be caught up on this point:

"Betting the turn accomplishes something. You gotta decide how often he calls. betting the flop accomplishes nothing. So i guess to me the flop is the big error."

But he evidently does not appear to appreciate that each of the options for hero on the flop and turn have calculable EVs, and that that is solely what should drive hero's decisions. Obviously betting the flop accomplishes many things, such as leveraging the range advantage, balancing the betting range, making villain folds better hands etc - which would all be well and good in a regular hand, but the high hand bonus EV in this hand of course changes everything and means that checking is the highest EV option and "accomplishes" the most as a result (because ultimately all that matters is EV). By the same token, betting the turn (as he indicated) is clearly an inferior, lower EV choice - despite the fact that hero might be able to get value from villain, which I can only assume is what he meant betting the turn accomplishes.

I think it's pretty interesting too, and notable that several forum users felt the high hand bonus EV was irrelevant to how the hand should be played. Absolutely, it would warp the game if taken further, like with the BBJ.

Yes exactly, the solution is simply for hero to check in order to realize 100% of the high hand bonus. What's also interesting is that there are high hand bonuses for every hand class at this casino, from any quads, to all of the x-high straight flushes, then to all of the individual (i.e. suited) royal flushes. They all have varying prizes, so it's interesting to consider at what size does the prize impacts strategy. From this hand it's clear that 200bb is enough to influence strategy very clearly, but I expect if it was only 100bb then it would be much closer.

while thats true, the pot is 20bb when u see the flop bc u iso'ed 7x lol. imagine the effect on like a 7bb pot or something

would look at like ev difference / size of pot or something

Damn yes of course you're right, I didn't appreciate that.

Wrong. The EVs are not calculable because you dont know V’s ranges or tendencies. They are estimable. So your flop decision can just be objectively shown as a punt. Your turn decision is based on assumptions, some of which are pretty bad if you blindly follow GTO. AND AGAIN, are based upon you BETTING THE FLOP, which was a punt.

I swear GTObrain is infuriating. The overconfidence is ridiculous. Calculable, get the **** out of here.

Well yeah dude poker is a game of imperfect information, estimated calculations is the best we can do, and solvers do that excellently based on reasonable human input.

However this does not prove that hero's flop decision can 'just be objectively shown to be a punt'. How do you come to that conclusion?

What assumptions do you think the turn decision is based on? Why are they bad if we "blindly follow GTO"?

You can huff and puff all you want, but it's meaningless unless you back up your claims with logical arguments. I'm genuinely interested to hear your views/input here.

Have you ever tried playing against a GTO bot? They are much tougher than human players. Game theory is the holy grail of poker, it's surprising to hear someone who is presumably an experienced player state otherwise.

why do u think betting the flop is horrible? im legitimately trying to follow along with what you're saying bc u seem to feel strongly about it lol

i mostly think the point of the thread was that the high hand bonus can turn the incentives of the game into something much different than base nl which he did a good job of proving. dunno why you'd berate him (i say this as someone that's berated him in other threads). is a good starting point for things like stand up game or progressive bonus or even just bounty hands, i dont think the actual hh matters much at all

Dont say duh. You confidently said youre right on the turn, and you confidently said i refuse to acknowledge the EV is “calculable”. Not only is it not, its not a mere matter of semantics, the fact its estimable and not calculable is the crux of my point

You ought to be able to tell me at this point so im not explaining again.

I like how you say duh poker is based on imperfect information but then dont know the assumptions gto is based on. This is extremely extremely extremely basic stuff. So basic that i advise against using a solver until you can answer it yourself.

Well then id recommend my previous posts.

Lmao.

I should probably expand on the “lmao” but its just more of your gtobrain arrogance, and youre just so arrogant that i dont feel incentivized to answer, but i oughta not match your arrogance with arrogance of my own, which i have done.

I like gto just fine, and i think your turn solves were well enough done. the EVs are in a range where a small change in Vs range or tendency would change the answer, so i think this is a reads based decision on the turn.

Also as ive already said, the more relevant question is what you’d do on the turn if you had checked the flop anyway, so I dont find the solution you gave to be a scalable solution to a more general question, which makes this exercise not very demonstrative to those involved, whereas the flop is both demonstrative and scalable. Dont semibluff with a high hand draw is a goid rule of thumb.

I understand what "polarized" means. The point I was making is that we either are or are not polarized. I don't understand what "fully polarized" means, unless we're going to debate that there are degrees of polarization ranging from almost none to completely.

I was also making the point that I'm not sure I agree your range is polarized here, unless you're saying you're ONLY betting the pure nuts, or pure bluffs. The fact that your hand isn't the pure nuts on the turn would seem top prove my point. If you're betting the nuts, the 2nd nuts, the 3rd nuts, etc, plus some draws, plus some air, you're not polarized, fully or otherwise.

Lastly, regardless of what the estimated EV is for all the possible actions on the turn, it's hard for the HJ to show up with a big hand on the turn when he limp-calls pre, we can account for 3 of the 5 biggest diamonds, and he likely would have raised pre if he had the other two.

I suppose we can massage the inputs to show the EV difference between betting small and betting large is minimal or zero, but if we're being reasonable, I think we'd see that betting small is going to increase the likelihood V calls, AND we see the river, whereas betting large is going to decrease that likelihood.

Further, betting small incentivizes V to raise with his strongest hands (mostly worse flushes, though a better flush isn't impossible here), whereas betting large doesn't, and makes it more likely he'll just smooth call with the strongest parts of his range, and fold the rest.

I'd think we'd want to bet small REGARDLESS of the high hand promotion, and any additional EV from it. But with the additional EV, I'd think betting small makes even MORE sense.

All the explanation of the solver outputs, and your defense of betting small - all totally unnecessary. I told you to bet small in my first comment, post 6 of this thread, not because a solver told me so, but because we really don't need a solver to figure all this out. I know, because I figured it out without using a solver. It's just logic and hand-reading filtered through experience.

If it makes you feel better, we can say that the EV is estimable. It really doesn't change anything. Even an approximation is better than nothing. Are you aware that calculating the turn EV involves thousands of calculations? And are you aware that game-theory based players who can best simulate the output of solvers are the biggest crushers in the modern game?

If you refuse to recognize the validity of solver EV solutions, how do you come to the conclusion that the flop bet is a punt? That's literally impossible to prove without using math, and it's perplexing that you won't give a clear answer on this despite being asked more than once.

I know how solvers work. You made the claim that the turn decision is "based on assumptions", so I'm just asking you to clarify what you believe those to be and why you believe it's bad if we "blindly follow GTO"? If you're not willing to answer simple questions, and are instead going to respond with strawmen and fist-waving, then there is little point in discussing these things.

What do you find arrogant in what I'm saying? I'm stating facts and solver output.

I very much doubt small changes in villain's range/tendencies are going to change the fact that checking will be the highest EV option on the turn in this hand. $22 is a huge boost in EV for checking, and in any case EVs are often very similar for various (reasonable) bet sizes and checking on the turn. This means that because checking is guaranteed to realize the full high hand bonus, it will always be the highest EV option. You can name any ranges and whatever bet sizes etc from flop to river that you want, I'll run the solve and post the output adjusted for the high hand bonus (using the math detailed above) and I guarantee you that checking will still be the highest EV option on the flop and the turn.

In the context of the solver on the turn at hero's decision point, there is no "reads-based decision". There is only the available betsizes/checking and their respective EVs to consider. I've played against villain many times; he's simply a regular low-stakes rec. Of course with all else being equal hero would be value betting the turn, but as indicated the high hand bonus makes checking the dominant strategy.

What I would do on the turn if I had checked the flop is to check again, because it would still be the highest EV option. Checking is the highest EV option on the turn and the flop regardless of anything else that happens in the hand, and that solution absolutely is scalable and glaringly obvious when comparing the EV of the various options available to hero on the flop/turn.

For sure, not semi-bluffing with a hand that can hit a high hand bonus is a good rule of thumb, but only when the bonus is large enough to have an impact on the EV of hero's decisions in such a way that checking is clearly better than betting.

How many people do you need to argue with in this forum before you'll be willing to consider the possibility that everyone else isn't the problem?

A hand's polarization can be determined by the action of the current and previous streets, because in general hero should be betting a polarized range from the flop forwards. If he checks at any point, for example on the flop, or on the turn after betting the flop, in theory his range becomes capped. In this hand, hero has iso-raised preflop, then bet on the flop and the turn. This means that his betting range is as polarized as possible. If hero had checked back the flop and then bet the turn, the betting range would still be polarized, but it would be less polarized than when he bets both flop and turn.

Polarized ranges do not contain only the literal nuts or air. They contain nutted hands and air (although the 'air' section involves semibluffs that obviously have x amount of equity on the flop/turn. As I mentioned in my last post, on this board the value section of hero's polarized betting range on the turn contains (amongst other things) flushes and sets. Your definition of a polarized range is just wrong I'm afraid. Doug Polk defines it here:

https://upswingpoker.com/polarized-vs-li...

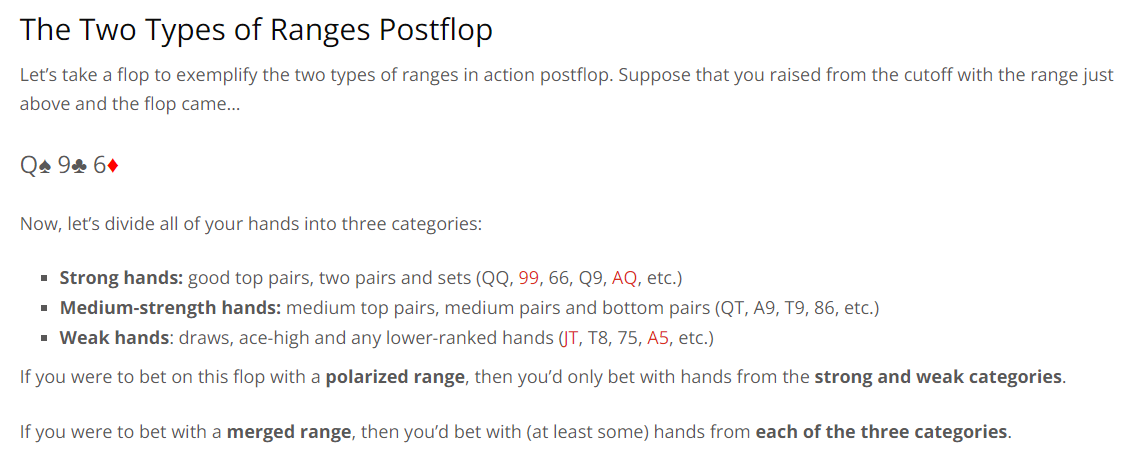
As you can see, the value section of polarized ranges includes even good top pairs.

Yes, hero is blocking quite a few of villain's value hands on the turn when the flush completes, and that fact is accounted for when the EV is calculated.

Betting smaller always increases the likelihood that villain calls/raises, but that doesn't necessarily increase the EV for hero. As you can see in the EV calculated in this hand on the turn, the EV is identical for betting 66% pot, 33% pot and checking:

Potsize is 175

Bet 830: EV 307
Bet 219: EV 321
Bet 116: EV 323
Bet 58: EV 323
Check: EV 323

This is quite common in solver output, especially on the flop and turn. In the case of this hand, we can see that overbetting the turn and jamming is losing EV compared to betting smaller/checking. I believe this is because overbetting the turn is suboptimal when the draw completes and villain has check-called flop and then checked to hero on the turn (usually overbets are good vs capped ranges, but villain isn't capped on this turn due to the draw completing). And going all-in for 830 is clearly a massive EV mistake that only a beginner would make, having turned the second nuts.

Yes, we would definitely want to bet on the smaller side (i.e. not an overbet+) on this turn regardless of the high hand bonus, simply because it's the highest EV option. With the high hand bonus in play, checking becomes easily the best option in terms of EV.

What you said in your original post on this thread was:

"It's a nut changing card. Just bet small, like 40% pot. The high hand overlay is just bonus EV".

This is missing the point and overlooking the fact that checking is clearly higher EV than any other option.

It's called a debate Einstein. It's what the smart kids were doing at school while you were busy sniffing glue

I'll put the O/U at 8.5.

We're at least half-way there.

Cool story bro.